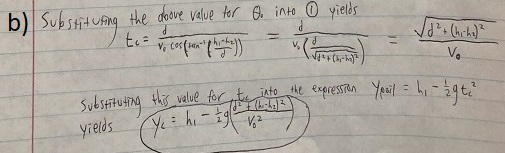Kinematics

This page describes the field of kinematics and presents some of the most important kinematic equations.

The Main Idea

The field of kinematics is the study of particle motion from a mathematical rather than physical point of view. More specifically, it studies the relationships between time and the position, velocity, and acceleration of particles, without regard for the forces that cause the acceleration. The equations that describe these relationships are called the kinematic equations, which are useful tools and the subject of most of this page. Often, these kinematic equations are used in combination with other branches of physics to predict the motion of an object at different points in time. For example, non-kinematic equations might be used to calculate the magnitude and direction of a force acting on a particle, as well as the acceleration that the particle undergoes as a result. These equations are not kinematic because they describe the causes and effects of forces. Then, kinematic equations might be used to calculate the position and speed of the particle as functions of time based on the acceleration found. These equations are kinematic because they relate position, velocity, acceleration, and time.

A Mathematical Model

Quantities of Kinematics

In order to understand the kinematic equations, one must be comfortable with the quantities they relate. The following are the quantities that the kinematic equations deal with:

Time ([math]\displaystyle{ t }[/math])
Position ([math]\displaystyle{ \vec{r} }[/math])
Velocity ([math]\displaystyle{ \vec{v} }[/math])
Acceleration ([math]\displaystyle{ \vec{a} }[/math])
Subsequent Time Derivatives of Position (less common)

Many of the values listed above depend on the Frame of Reference, or coordinate system, chosen because the reference frame defines which points in space and time are considered x = 0, y = 0, z = 0, and t = 0. The following kinematic equations assume a reference frame of arbitrary spatial origin but with time t=0 defined as the time at which the particle is in its initial position. This assumption is made to avoid having to write [math]\displaystyle{ \Delta t }[/math] instead of [math]\displaystyle{ t }[/math] everywhere the time variable occurs, and because other definitions of t=0 are rarely used.

Subscripts of i and f respectively denote the initial and final values of these quantities over some time interval, the beginning of which is t=0.

Some of the kinematic equations make use of the average velocity ([math]\displaystyle{ \vec{v}_{avg} }[/math]). The instantaneous velocity often changes as a function of time like position, but over a given time interval, it has an average value. The average velocity is given by the displacement over a time interval divided by the duration of that time interval. (See Derivation of Average Velocity.) If the velocity is constant over time, that velocity is the average velocity.

The Kinematic Equations

[math]\displaystyle{ \vec{r}_f = \vec{r}_i + \vec{v}_{avg} \cdot t }[/math]
[math]\displaystyle{ \vec{v}_f = \vec{v}_i + \vec{a}_{avg} \cdot t }[/math] (typically this equation is used in situations of constant acceleration, where [math]\displaystyle{ \vec{a}_{avg} }[/math] is that constant acceleration.)
[math]\displaystyle{ \vec{v}_{avg} = \frac{\vec{v}_i + \vec{v}_f}{2} }[/math] (assumes constant acceleration)
[math]\displaystyle{ \vec{r}_f = \frac{1}{2} \vec{a} \cdot t^2 + \vec{v}_i \cdot t + \vec{r}_i }[/math] (assumes constant acceleration)
[math]\displaystyle{ v_f^2 = v_i^2 + 2 \vec{a}(\vec{r}_f - \vec{r}_i) }[/math] (assumes constant acceleration)

These are the standard kinematics equations that are often used to model motion in physics. That said, other equations do exist (for example, calculus-based versions of the above equations that do not assume constant acceleration, as well equations that refer to the higher time derivatives of position such as jerk).

Usually when kinematic equations are used to solve problems, one-dimensional versions are used so that vector addition doesn't need to be performed. For example, equation 3 can be decomposed into x and y components as follows:

[math]\displaystyle{ x_f = \frac{1}{2} a_x \cdot t^2 + v_{x, i} \cdot t + x_i }[/math]

[math]\displaystyle{ y_f = \frac{1}{2} a_y \cdot t^2 + v_{y, i} \cdot t + y_i }[/math] hu These equations are best derived with calculus, but it is possible to do so without its use. First, we begin with the definition

[math]\displaystyle{ v_{avg} = \frac{\vec{r}_f - \vec{r}_i}{t} }[/math]

from which (1) follows immediately. Now, let us assume constant force, which implies constant acceleration (in the case of constant mass, which we will assume in most cases for this course) through the expression of Newton's Second Law: the Momentum Principle

[math]\displaystyle{ \vec{F} = m\vec{a} }[/math]

Since we also have acceleration by definition as

[math]\displaystyle{ \vec{a} = \frac{\vec{v}_f - \vec{v}_i}{t} }[/math]

Then similar to (1), (2) follows from rearrangement. Furthermore, (3) is the definition of an average. Now we come to the interesting equations. Expanding (1) using (3) gives

[math]\displaystyle{ \vec{r}_f = \vec{r}_i + \frac{\vec{v}_f +\vec{v}_i}{2}t }[/math]

Now, we do a rearrangement trick:

[math]\displaystyle{ \vec{r}_f = \vec{r}_i + \frac{\vec{v}_f-\vec{v}_i}{2}t + \vec{v}_i \cdot t = \vec{r}_i + \vec{v}_i \cdot t + \frac{\vec{a}}{2}t^2 }[/math]

Which is (4). Now, we rearrange this a little to get

[math]\displaystyle{ \vec{r}_f - \vec{r}_i = \vec{v}_i \cdot t + \frac{\vec{a}}{2}t^2 }[/math]

[math]\displaystyle{ 2(\vec{r}_f - \vec{r}_i)\cdot \vec{a} = 2\cdot\vec{a}\cdot\vec{v}_i\cdot t + \vec{a}^2\cdot t^2 }[/math]

Substituting in the definition of [math]\displaystyle{ \vec{a} }[/math] for only the right side gives

[math]\displaystyle{ 2(\vec{r}_f - \vec{r}_i)\cdot \vec{a} = 2\cdot\frac{\vec{v}_f-\vec{v}_i}{t}\cdot\vec{v}_i\cdot t + (\frac{{v}_f-\vec{v}_i}{t})^2\cdot t^2 }[/math]

[math]\displaystyle{ 2(\vec{r}_f - \vec{r}_i)\cdot \vec{a} = 2\vec{v}_f\cdot\vec{v}_i -2\vec{v}_i^2 + \vec{v}_f^2 - 2\vec{v}_f\cdot\vec{v}_i + \vec{v}_i^2 }[/math]

[math]\displaystyle{ 2(\vec{r}_f - \vec{r}_i)\cdot \vec{a} + \vec{v}_i^2 = \vec{v}_f^2 }[/math]

Thus we have arrived at equation (5). One may justly ask why we chose to jump through these hoops, and the answer is, for (4) and (5) respectively, calculus and the Work/Energy theorem. That being said, it is instructive to see that under the assumption of constant force, these equations may be derived without calculus. Indeed, the same may be done for cases of constant jerk, snap, crackle, or pop, but it is more complicated and essentially useless, so we won't bother with it here.

Examples

1. (Simple)

An airplane begins at rest and accelerates down a runway at a constant [math]\displaystyle{ 3.20 \; \frac{m}{s^2} }[/math] for [math]\displaystyle{ 32.8 \; s }[/math] until is finally lifts off the ground. Determine a) the distance traveled before takeoff and b) the final velocity of the airplane.

Solution

a) The distance traveled can be determined with the following equation:

[math]\displaystyle{ \vec{r}_f = \frac{1}{2} \vec{a} \cdot t^2 + \vec{v}_i \cdot t + \vec{r}_i }[/math]

A one-dimensional version can be used because we are only concerned with motion along one axis:

[math]\displaystyle{ x_f = \frac{1}{2} a_x \cdot t^2 + v_{x, i} \cdot t + x_i }[/math]

The initial velocity of the plane is zero. If we define the origin to be the initial position of the plane, its initial position will be 0 as well, and the final position will be the same as distance traveled. Time t=0 is the instant the plane begins to accelerate.

[math]\displaystyle{ x_f = \frac{1}{2} (3.20 \; \frac{m}{s^2}) \cdot (32.8 \; s)^2 = 1720 \; m }[/math]

b) The final velocity can be determined with the following equation:

[math]\displaystyle{ \vec{v}_f = \vec{v}_i + \vec{a} \cdot t }[/math]

Again, let use use a one-dimensional version:

[math]\displaystyle{ v_{x, f} = v_{x, i} + a_x \cdot t }[/math]

[math]\displaystyle{ v_{x, f} = (3.20 \; \frac{m}{s^2}) \cdot (32.8 \; s) = 105 \; \frac{m}{s} }[/math]

The final velocity can alternatively be found using the equation [math]\displaystyle{ v_{x, f}^2 = v_{x, i}^2 + 2 a_x (x_f - x_i) }[/math]; all variables are known from the given information and the distance found above except [math]\displaystyle{ v_{x, f} }[/math].

2. (Medium)

A person throws a stone at an initial angle θ = 45[math]\displaystyle{ ^\circ }[/math] from the horizontal with an initial speed of v =20m/s. The point of release of the stone is at a height d=2m above the ground. You may neglect air resistance.

a) How long does it take the stone to reach the highest point of its trajectory?

b) What was the maximum vertical displacement of the stone? Ignore air resistance.

c) What horizontal distance away does the stone land?

Let us call the origin the point on the ground directly below the point from which the stone is released, and let us define +y as upwards and +x as horizontally in the direction of the stone's travel. t=0 is the instant in time at which the stone is released.

a) At the highest point of the stone's trajectory, its vertical velocity is 0; the stone is moving entirely horizontally. Let us use this fact to determine how long the stone takes to get there, along with the following equation:

[math]\displaystyle{ v_{y, f} = v_{y, i} + a_y * t }[/math]

[math]\displaystyle{ 0 = 20\sin45 - 9.8 * t }[/math]

Solving for [math]\displaystyle{ t }[/math] yields [math]\displaystyle{ t = 1.44 }[/math]s.

b) Now that we know how long it takes the stone to reach its highest point, we can use the following equation to find what that highest point its:

[math]\displaystyle{ y_f = \frac{1}{2} a_y * t^2 + v_{y, i} * t + y_i }[/math]

[math]\displaystyle{ y_f = \frac{1}{2} (-9.8) * (1.44)^2 + 20\sin45 * 1.44 + 2 = 12.2 }[/math]m

The maximum height can alternatively be found using the equation [math]\displaystyle{ v_{y, f}^2 = v_{y, i}^2 + 2 a_y (y_f - y_i) }[/math]; all variables are known from the given information except [math]\displaystyle{ y_f }[/math].

c) The stone lands at height [math]\displaystyle{ y=0 }[/math], so let us use the following equation to see how long it takes the stone to get there. We need this information because then we know how much time the stone has to move in the +x direction before it hits the ground.

[math]\displaystyle{ y_f = \frac{1}{2} a_y * t^2 + v_{y, i} * t + y_i }[/math]

[math]\displaystyle{ 0 = \frac{1}{2} (-9.8) * t^2 + 20\sin45 * t + 2 }[/math]

The quadratic equation must be used to solve for t:

[math]\displaystyle{ t = \frac{-b\pm\sqrt{b^2-4ac}}{2a} }[/math] (Note: here a, b, and c are the coefficients on the quadratic equation above. Do not confuse this "a" with the "a" signifying acceleration.)

[math]\displaystyle{ t = \frac{-20\sin45 \pm\sqrt{(20\sin45)^2-4 * \frac{1}{2}(-9.8) * 2}}{2 * \frac{1}{2}(-9.8)} }[/math]

[math]\displaystyle{ t = -.135, 3.02 }[/math]s.

There are two solutions because there are two times at which the ball is at the height y=0 using its parabolic trajectory. The first time, [math]\displaystyle{ t = -.135 }[/math]s, represents the time the ball would have been at ground height if it had been traveling along its parabolic path before time t=0. At this point in time, it would have been on its way up. The second time, [math]\displaystyle{ t = 3.02 }[/math]s, represents the time that the ball actually hits the ground on its way down. This is the time we are interested in.

Note that if the stone were to land at the same height from which it was launched, the time of flight would simply be twice the time it takes to reach the highest point. This is not the case for this problem because the stone is launched from 2m above the ground but lands on the ground.

Now we must use this time with a second kinematic equation involving the x coordinate of the ball to determine the horizontal distance it traveled while it was in the air. The equation we can use is:

[math]\displaystyle{ x_f = x_i + v_{x, avg} * t }[/math]

[math]\displaystyle{ v_{x, avg} }[/math] is simply the initial horizontal velocity the ball was thrown with because no forces act on the ball in the x direction; [math]\displaystyle{ v_x }[/math] is constant.

[math]\displaystyle{ x_f = 20\cos45 * 3.02 = 42.73 }[/math]m

3. (Difficult)

A person is standing on a ladder holding a pail. The person releases the pail from rest at a height [math]\displaystyle{ h1 }[/math] above the ground. A second person standing a horizontal distance [math]\displaystyle{ d }[/math] from the pail aims and throws a ball the instant the pail is released in order to hit the pail. The ball is thrown from a height [math]\displaystyle{ h2 }[/math] above the ground with initial speed [math]\displaystyle{ v_0 }[/math]. Ignore air resistance.

a) At what angle [math]\displaystyle{ \Theta_0 }[/math] above the horizontal should the ball be thrown in order to hit the pail? Express your answer in terms of the other variables given.

b) How high above the ground does the collision occur?

For more practice with Kinematics, try the examples on the Projectile Motion page or the first few on the Acceleration page.

Connectedness

Application: Infrastructure/ Civil Engineering

The infrastructure on which vehicles travel must be built to accommodate their ability to speed up and slow down. For example, turn lanes should be longer where the speed limit is greater because a vehicle traveling at greater speeds needs more space to come to a stop. With knowledge of a vehicle's initial speed and ability to decelerate, kinematic equations can be used to determine the minimum length of a turn lane. Similarly, runways must be long enough to allow panes to reach takeoff speed. If the acceleration of a plane and the necessary speed for takeoff are known, kinematic equations can be used to calculate the minimum length of a runway.

Application: Accident Investigation

Imagine that a driver is moving down a straight road when suddenly another car backs out of a parking space onto the road in front of them. The driver slams on the brakes but does not have enough space to come to a stop and collides with the car backing out. Normally the driver of the parked vehicle would be considered at fault because you are responsible for checking behind you before you back out. However, an investigator may look at the marks left on the road by the skidding tires of the braking vehicle. Using the length of the marks and a kinematic equation, the investigator can determine the initial speed of the braking vehicle, and the driver may be held responsible for the accident if they were speeding.

Scenario: Projectile Motion

Because objects near the surface of the earth undergo constant acceleration, kinematic equations can be used to describe their motion.

History

Although humans have questioned the nature of motion for thousands of years, Isaac Newton is credited for the advancement of most topics in kinematics. In 1669, Newton was appointed professor of mathematics at Trinity College. In January 1672, he was elected to the Royal Society, a loose organizations of scientists and intellectuals. Shortly thereafter, he presented a paper detailing his discoveries in optics, and developed a rivalry with the scientist Robert Hooke, who harshly criticized Newton's research. This rivalry would percolate throughout the 1670s, as Newton continued to work out the mathematics of gravity, and would again flare up in the mid 1680s when Newton finally published his work, some of which Hooke felt had been stolen from him.

Newton's research was organized into a three-volume book, the Philosophiae Naturalis Principia Mathematica ("Mathematical Principles of Natural Philosophy"), known to posterity as the Principia Mathematica. It set forth Newton's three laws of motion, and proceeded to set forth the theory of gravitation, and back it up with rigorous mathematical proofs. Although the theory had many detractors at first, the scientific community would ultimately embrace it, and the Newtonian world-view would dominate physics until the 20th century.

http://web.mit.edu/8.01t/www/materials/modules/chapter04.pdf

http://www.sparknotes.com/biography/newton/summary.html

http://www.physicsclassroom.com/class/1DKin/Lesson-6/Kinematic-Equations