Lorentz Transformations
The Lorentz Transformation is a transformation that allows one to shift between different coordinate systems. Namely, it allows one to transform the cartesian coordinate system of a stationary reference frame to another cartesian coordinate system of a reference frame that is moving with constant velocity [math]\displaystyle{ v }[/math] with respect to the stationary reference frame. It is named after Hendrik Antoon Lorentz, who derived the transformation in 1904 while working on developing the theory of electrodynamics for moving bodies (2). The transformation is as follows
[math]\displaystyle{ \begin{align}x'&=\gamma(x-vt)\\ y'&=y\\ z'&=z\\ t'&=\gamma(t-\frac{xv}{c^2})\end{align} }[/math]
where [math]\displaystyle{ \gamma=\frac{1}{\sqrt{1-v^2/c^2}} }[/math].
The Lorentz Transformation can be considered a more general formulation of of the ideas of time dilation and length contraction as it gives not just the changes in length and in time when going from one reference frame to another, but rather it gives the full coordinates of objects in space and time when going from one reference frame to another. Because it is a more general formulation, Einstein's equations for time dilation and length contraction can be derived from the Lorentz Transformation (shown in exercise 2.2). Going further, we can also use the Lorentz Transformation to relate observed speeds in different reference frames, which is done by calculating differentials from the Lorentz Transformation (shown in exercise 2.3)(2).
Derivation of the Lorentz Transformation
The following derivation is from (4).
To derive the Lorentz Transformation, we start by considering the movement of light from two difference reference frames. Our unprimed reference frame [math]\displaystyle{ (x,y,z,t) }[/math] will be referred to as the stationary reference frame, and our primed reference frame [math]\displaystyle{ (x',y',z't') }[/math] will be referred to as the moving reference frame. We will define the relationship between the two reference frames such that an observer in the stationary reference frame see another observer in the moving reference frame moving along the positive x-axis with speed [math]\displaystyle{ v }[/math].
Now imagine that the movement of a light wave is observed from both reference frames for some length of time. In the stationary reference frame, we can express the length of this path in two ways as shown below.
[math]\displaystyle{ \begin{align}&l=c(t_2-t_1)\\&l=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2+(z_2-z_1)^2}\end{align} }[/math]
Now we will rearrange the above expressions for [math]\displaystyle{ l }[/math] to get.
[math]\displaystyle{ \begin{align}&l^2-l^2=0\\&c^2(t_2-t_1)^2-(x_2-x_1)^2-(y_2-y_1)^2-(z_2-z_1)^2=0\end{align} }[/math]
We can now do the same procedure for the moving reference frame, and we get the expressions below
[math]\displaystyle{ \begin{align}&l'=c(t_2'-t_1')\\&l'=\sqrt{(x_2'-x_1')^2+(y_2'-y_1')^2+(z_2'-z_1')^2}\\&l'^2-l'^2=0\\&c^2(t_2'-t_1')^2-(x_2'-x_1')^2-(y_2'-y_1')^2-(z_2'-z_1')^2=0\end{align} }[/math]
Now we have expressions in both of our reference frames that are equal to zero, so we can set them equal to each other to determine the relationship between our difference coordinate systems.
[math]\displaystyle{ c^2(t_2'-t_1')^2-(x_2'-x_1')^2-(y_2'-y_1')^2-(z_2'-z_1')^2=c^2(t_2-t_1)^2-(x_2-x_1)^2-(y_2-y_1)^2-(z_2-z_1)^2 }[/math]
Using a clever trick, we can simplify the above equation greatly. We will let the motion of the light wave be purely along the [math]\displaystyle{ x,x' }[/math] axis. Remember that we can set up our coordinate axes however is convenient for us when solving problems, so this trick is not requiring any further assumptions. If the light wave's motion is purely along the [math]\displaystyle{ x,x' }[/math] axis, then we can simplify the above equation to the following.
[math]\displaystyle{ c^2(t_2'-t_1')^2-(x_2'-x_1')^2=c^2(t_2-t_1)^2-(x_2-x_1)^2 }[/math]
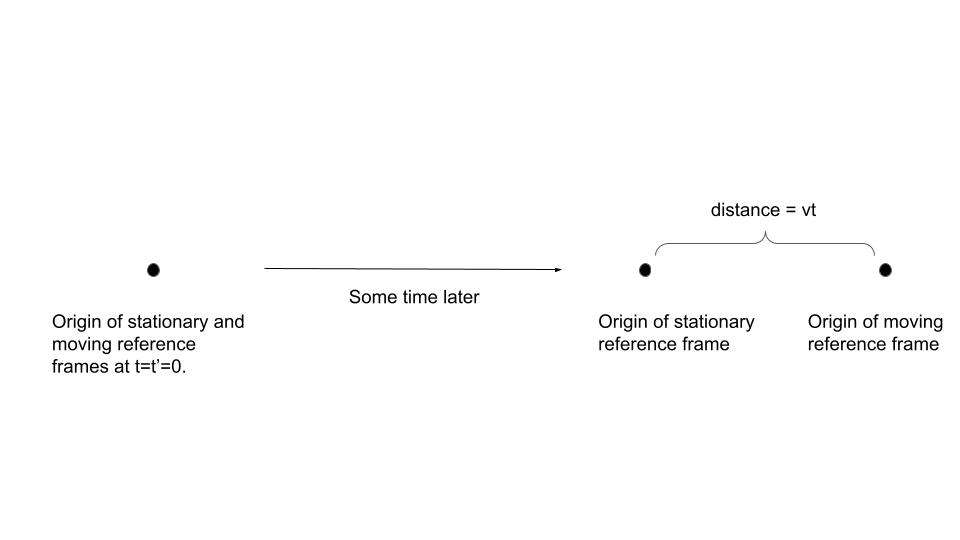
We can further reduce our equation by letting the light wave start at the origin of both coordinate systems (i.e. [math]\displaystyle{ t_1=t_1'=0 }[/math] and [math]\displaystyle{ x_1=x_1'=0 }[/math]). The figure below illustrates the initial overlap of the origins of our coordinate systems.
Now we can rewrite the above equation as.
[math]\displaystyle{ c^2t'^2-x'^2=c^2t^2-x^2 }[/math]
The general solution solved for the moving reference frame to the above equation is
[math]\displaystyle{ x'=xcosh\;\alpha+ctsinh\;\alpha\;\;\;\;\;\;\;\;\;\;\; ct'=xsinh\;\alpha+ctcosh\;\alpha }[/math]
where [math]\displaystyle{ \alpha\in\mathbb{R} }[/math] (this is shown to be the general solution to the above equation in section 1.2). Our next step in deriving the Lorentz Transformation is to find expressions for [math]\displaystyle{ sinh\alpha }[/math] and [math]\displaystyle{ cosh\alpha }[/math]. This can be done by considering another thought experiment: consider tracking the origin of the stationary reference frame from the perspective of the moving reference frame. This simplifies the above general solution to the following since [math]\displaystyle{ x=0 }[/math].
[math]\displaystyle{ x'=ctsinh\;\alpha\;\;\;\;\;ct'=ctcosh\;\alpha }[/math]
Now we can find expressions for [math]\displaystyle{ sinh\;\alpha }[/math] and [math]\displaystyle{ cosh\;\alpha }[/math], which we can then plug back into our general equation for [math]\displaystyle{ x' }[/math] and [math]\displaystyle{ ct' }[/math] to get the Lorentz Transformation. Note: to find expressions for [math]\displaystyle{ sinh\;\alpha }[/math] and [math]\displaystyle{ cosh\;\alpha }[/math], we use the identities stated in section 1.1.
[math]\displaystyle{ \frac{x'}{ct'}=\frac{-v}{c}=tanh\;\alpha\;\;\;\; \Rightarrow \;\;\;\;sinh\;\alpha=\frac{-v/c}{\sqrt{1-v^2/c^2}} \;\;\;\; cosh\;\alpha=\frac{1}{\sqrt{1-v^2/c^2}} }[/math]
Here, [math]\displaystyle{ \frac{x'}{t'}=-v }[/math] because in the moving refernece frame, the origin of the stationary reference frame is moving in the negative [math]\displaystyle{ x }[/math] direction with speed [math]\displaystyle{ v }[/math]. Thus, the [math]\displaystyle{ x' }[/math] position of the origin of the stationary reference frame from the perspective of the moving reference frame is expressed as [math]\displaystyle{ x'=-vt' }[/math].
[math]\displaystyle{ x'=xcosh\;\alpha+ctsinh\;\alpha\;\; \Rightarrow \;\; x\frac{1}{\sqrt{1-v^2/c^2}}+ct\frac{-v/c}{\sqrt{1-v^2/c^2}}=\frac{x-vt}{\sqrt{1-v^2/c^2}}=\gamma(x-vt) }[/math]
[math]\displaystyle{ ct'=xsinh\;\alpha+ctcosh\;\alpha=x\frac{-v/c}{\sqrt{1-v^2/c^2}}+ct\frac{1}{\sqrt{1-v^2/c^2}}=\frac{ct-xv/c}{\sqrt{1-v^2/c^2}}=c\gamma(t-xv/c^2) }[/math]
[math]\displaystyle{ \Rightarrow \;\;\;\;x'=\gamma(x-vt)\;\;\;\; t'=\gamma(t-xv/c^2) }[/math]
The [math]\displaystyle{ y }[/math] and [math]\displaystyle{ z }[/math] components of the Lorentz Transformation are simply [math]\displaystyle{ y'=y }[/math] and [math]\displaystyle{ z'=z }[/math]. This is because in our derivation for the [math]\displaystyle{ x }[/math] and [math]\displaystyle{ t }[/math] components of the transformation, we had to assume that the motion of our imaginary light wave was purely along the [math]\displaystyle{ x }[/math] axis in the stationary reference frame, and purely along the [math]\displaystyle{ x' }[/math] axis in the moving reference frame. So the [math]\displaystyle{ y,z,y',z' }[/math] coordinates of the light wave do not change over time. Also, since we assumed in our derivation that the origins of our coordinate systems coincide, the initial [math]\displaystyle{ y,y' }[/math] and [math]\displaystyle{ z,z' }[/math] coordinates must agree with each other. Thus, [math]\displaystyle{ y'=y }[/math] and [math]\displaystyle{ z'=z }[/math].
We have now arrived at the full Lorentz Transformation:
[math]\displaystyle{ \begin{align}x'&=\gamma(x-vt)\\ y'&=y\\ z'&=z\\ t'&=\gamma(t-\frac{xv}{c^2})\end{align} }[/math]
where [math]\displaystyle{ \gamma=\frac{1}{\sqrt{1-v^2/c^2}} }[/math].
A similar derivation yields an alternate formulation of the Lorentz Transformation that transforms the primed reference frame into the unprimed one. It is as follows.
[math]\displaystyle{ \begin{align}x&=\gamma(x'+vt')\\ y&=y'\\ z&=z'\\ t&=\gamma(t'+\frac{x'v}{c^2})\end{align} }[/math]
This is also sometimes referred to as the Inverse Lorentz Transformation, and it is equivalent to the regular Lorentz Transformation except that the relative motion between the reference frames is switched (i.e. here we consider the primed reference frame moving away from the unprimed one in the positive [math]\displaystyle{ x }[/math] direction, where as for the previous derivation we considered the unprimed reference frame moving away from the primed one in the negative [math]\displaystyle{ x' }[/math] direction).
Hyperbolic Trigonometric Identities
[math]\displaystyle{ \begin{align} &cosh^2\;\alpha-sinh^2\;\alpha=1\\ &sinh\;\alpha=\frac{tanh\;\alpha}{\sqrt{1-tanh^2\;\alpha}}\\ &cosh\;\alpha=\frac{1}{\sqrt{1-tanh^2\;\alpha}} \end{align} }[/math]
Verification of the General Solution
Our general solution to [math]\displaystyle{ c^2t'^2-x'^2=c^2t^2-x^2 }[/math] is [math]\displaystyle{ x'=xcosh\;\alpha+ctsinh\;\alpha\;\;ct'=ctcosh\;\alpha+xsinh\;\alpha }[/math]. We will verify this via direct substitution.
[math]\displaystyle{ ct'^2-x'^2=ct^2-x^2 }[/math]
We will plug in [math]\displaystyle{ x'=xcosh\;\alpha+ctsinh\;\alpha\;\;ct'=ctcosh\;\alpha+xsinh\;\alpha }[/math] to the left hand side of the above equation and show that it is equal to the right hand side.
[math]\displaystyle{ \begin{align} (ctcosh\;\alpha+xsinh\;\alpha)^2-(xcosh\;\alpha+ctsinh\;\alpha)^2&=c^2t^2cosh^2\;\alpha+x^2sinh^2\;\alpha+2ctxcosh\;\alpha\;sinh\;\alpha-(x^2cosh^2\;\alpha+c^2t^2sinh^2\;\alpha+2ctxcosh\;\alpha\;sinh\;\alpha)\\ &=(c^2t^2cosh^2\;\alpha-c^2t^2sinh^2\;\alpha)-(x^2cosh^2\;\alpha-x^2sinh^2\;\alpha)+(2ctxcosh\;\alpha\;sinh\;\alpha-2ctxcosh\;\alpha\;sinh\;\alpha)\\ &=c^2t^2(cosh^2\;\alpha-sinh^2\;\alpha)-x^2(cosh^2\;\alpha-sinh^2\;\alpha)\\ &=c^2t^2-x^2 \end{align} }[/math]
Therefore [math]\displaystyle{ x'=xcosh\;\alpha+ctsinh\;\alpha\;\;ct'=ctcosh\;\alpha+xsinh\;\alpha }[/math] is the general solution to [math]\displaystyle{ c^2t'^2-x'^2=c^2t^2-x^2 }[/math].
A Mathematical Model
There are several equations related to the Lorentz Transformation which can be useful in special relativity. First and foremost, there is the standard Lorentz Transformation.
[math]\displaystyle{ \begin{align} x'&=\gamma(x-vt)\\ y'&=y\\ z'&=z\\ t'&=\gamma(t-vx/c^2) \end{align} }[/math]
We also have the alternate formulation of the Lorentz Transformation mentioned above.
[math]\displaystyle{ \begin{align} x&=\gamma(x'+vt')\\ y&=y'\\ z&=z'\\ t&=\gamma(t'+vx'/c^2) \end{align} }[/math]
We also have equations relating how intervals of time and length expand and contract when viewed in different reference frames; these are the Einstein Time Dilation and Length Contraction equations derived in section 2.2
[math]\displaystyle{ \begin{align} \Delta t'&=\gamma\Delta t\\ L'&=L/\gamma \end{align} }[/math]
Lastly, we have the velocity transformation equations, which are derived in section 2.3.
[math]\displaystyle{ \begin{align} v_x'&=\frac{v_x-u}{1-v_xu/c^2}\\ v_y'&=\frac{v_y}{\gamma(1-v_xu/c^2)}\\ v_z'&=\frac{v_z}{\gamma(1-v_xu/c^2)} \end{align} }[/math]
In the above equations, [math]\displaystyle{ u }[/math] is the relative speed between our two reference frames (what was formerly denoted as [math]\displaystyle{ v }[/math]).
A Computational Model
Visualization of a wheel rotating at relativistic speeds by Thierry Dugnolle (also shown to the right)

Examples
Simple
Given that an event happens at location [math]\displaystyle{ (x,y,z)=(1.0\;m,5.0\;m,3.0\;m) }[/math] at time [math]\displaystyle{ t=1.2\;s }[/math] in a stationary reference frame, what are the coordinates of the event in a reference frame that moves with speed [math]\displaystyle{ v=.75\;c }[/math] in the positive [math]\displaystyle{ x }[/math] direction from the perspective of the stationary reference frame? At what time in this moving reference frame will the event happen? Assume that the origins of the two reference frames coincide at [math]\displaystyle{ t=t'=0 }[/math].
Solution:
We are given the full coordinates in space and time for the event in the unprimed, stationary, reference frame. So we can get the coordinates of the event in space and time in the primed, moving, reference frame simply by applying the Lorentz Transformation to each coordinate.
[math]\displaystyle{ x'=\gamma(x-vt)=\frac{1.0\;m-.75c(1.2\;s)}{\sqrt{1-.75^2c^2/c^2}}=\frac{1.0\;m-.75c(1.2\;s)}{\sqrt{1-.75^2}}=-4.1\times 10^8 \;m }[/math]
[math]\displaystyle{ y'=y=5.0\;m }[/math]
[math]\displaystyle{ z'=z=3.0\;m }[/math]
[math]\displaystyle{ t'=\gamma(t-vx/c^2)=\frac{1.2\;s-.75c(1.0\;m)/c^2}{\sqrt{1-.75^2c^2/c^2}}=\frac{1.2\;s-.75(1.0\;m)/c}{\sqrt{1-.75^2}}=1.8\;s }[/math]
Middling
Derive Einstein's equations for time dilation ([math]\displaystyle{ \Delta t'=\gamma \Delta t }[/math], where ) and length contraction ([math]\displaystyle{ L'=L/\gamma }[/math]) from the Lorentz Transformation, where [math]\displaystyle{ \Delta t }[/math] and [math]\displaystyle{ L }[/math] are both measured in a stationary reference frame.
Solution:
[math]\displaystyle{ \begin{align} \Delta t'&=t'_2-t'_1\\ &=\gamma(t_2-vx/c^2)-\gamma(t_1-vx/c^2)\;\;\; \textnormal{here we are just considering differences in time intervals, so we assume that}\; x\; \textnormal{position does not change}\\ &=\gamma(t_2-t_1-vx/c^2+vx/c^2)\\ &=\gamma(t_2-t_1)\\ &=\gamma\Delta t\\ \Rightarrow \Delta t'&=\gamma\Delta t \end{align} }[/math]
Consider a rod of length [math]\displaystyle{ L }[/math] in a stationary reference frame and a clock in a moving reference frame moving with speed [math]\displaystyle{ v }[/math] down the length of the rod. Here, [math]\displaystyle{ L'=v\Delta t }[/math] and [math]\displaystyle{ L=v\Delta t' }[/math]. Using the above result, we have the following (1).
[math]\displaystyle{ \frac{L'}{L}=\frac{v\Delta t}{v\Delta t'}=1/\gamma }[/math]
[math]\displaystyle{ \Rightarrow\; L'=L/\gamma }[/math]
Difficult
Show that [math]\displaystyle{ v_x'=\frac{v_x-u}{1-v_xu/c^2} }[/math], [math]\displaystyle{ v_y'=\frac{v_y}{\gamma(1-v_xu/c^2)} }[/math], and [math]\displaystyle{ v_z'=\frac{v_z}{\gamma(1-v_xu/c^2)} }[/math] are the velocity transformation formulae between reference frames where [math]\displaystyle{ u }[/math] is the speed of the moving reference frame from the perspective of the stationary reference frame (this was formerly [math]\displaystyle{ v }[/math] in our derivation of the Lorentz Transformation).
Solution (3):
We begin (as stated in the introduction) by calculating the differentials of the Lorentz Transformation, which is shown below.
[math]\displaystyle{ x'=\gamma(x-ut)\;\;\;\;\rightarrow\;\;\;\; dx'=\gamma(dx-udt) }[/math]
[math]\displaystyle{ y'=y\;\;\;\;\rightarrow\;\;\;\; dy'=dy }[/math]
[math]\displaystyle{ z'=z\;\;\;\;\rightarrow\;\;\;\; dz'=dz }[/math]
[math]\displaystyle{ t'=\gamma(t-ux/c^2)\;\;\;\;\rightarrow\;\;\;\;dt'=\gamma(dt-udx/c^2) }[/math]
Now, we use the above differentials to determine formulae relating speeds between different reference frames.
[math]\displaystyle{ v_x'=\frac{dx'}{dt'}=\frac{\gamma(dx-udt)}{\gamma(dt-udx/c^2)}=\frac{dx-udt}{dt-udx/c^2}=\frac{v_x-u}{1-v_xu/c^2} }[/math]
[math]\displaystyle{ v_y'=\frac{dy'}{dt'}=\frac{dy}{\gamma(dt-udx/c^2)}=\frac{v_y}{\gamma(1-v_xu/c^2)} }[/math]
[math]\displaystyle{ v_z'=\frac{dz'}{dt'}=\frac{dz}{\gamma(dt-udx/c^2)}=\frac{v_z}{\gamma(1-v_xu/c^2)} }[/math]
Connectedness
The Lorentz Transformation is an essential part of the Special Theory of Relativity, a subject I find to be fascinating. My major is physics, so the topic of the Lorentz Transformation appears in several different areas of my major. Namely, it shows up whenever objects are moving very fast and relativistic effects need to be taken into account. An interesting industrial application of the Lorentz Transformation and Special Relativity is the operations of satellites. Because of the incredibly fast orbital speeds of satellites, time dilation must be taken into account when using satellites for things such as GPS (5).
History
The Lorentz Transformation was first derived not by Lorentz, but rather by Woldemar Voigt in a paper he published in 1897 (W. Voigt, Theorie des Lichts für bewegte Medien, Nachrichten von der Königlichen Gesellschaft der Wissenschaften und der Georg–Augusts–Universität zu Göttingen, No. 8, 11. Mai 1887). In this paper, Voigt took an alternative approach to the already derived Doppler Effect in which he postulated that the wave equation should take on the same form in both the stationary and moving reference frames (a postulate for which he gave no reason). This postulate is equivalent to stating that the propagation velocity of the wave must be invariant under transformations between reference frames that are moving with constant velocity relative to one another (2).
For reasons not known, Hendrik Antoon Lorentz appeared to be inspired by this transformation. He rederived it in his 1904 paper (H. A. Lorentz, Electromagnetic phenomena in a system moving with any velocity smaller than that of light, Proceedings Acad. Sc. Amsterdam 6 (1904) 809) in which he investigated the electrodynamics of moving bodies. The transformation was eventually named after Lorentz in 1906 by Jules Henri Poincaré (2).
See also
Einstein's Theory of Special Relativity
Further reading
Further explanation and example problems
External links
YouTube video series on special relativity and the Lorentz Transformation by Henry Reich here
References
(1) David Halliday, Robert Resnick, Jearl Walker (2010), Fundamentals of Physics
(2) Engelhardt, W.W.. (2013). On the Origin of the Lorentz Transformation.
(3) Kenneth S. Krane, (2019), Modern Physics
(4) L. D. Landau, E. M. Liftshitz (1967), The Classical Theory of Fields
(5) https://www.space.com/36273-theory-special-relativity.html