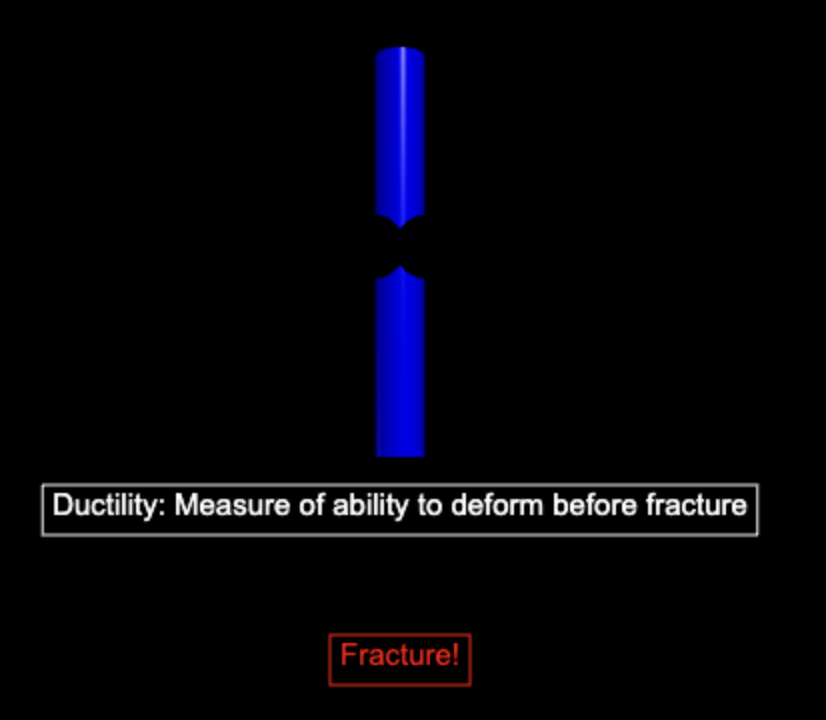
Ductility

Edited by Irene Dumitriu (Spring 2024)

The Main Idea

Ductility is a solids ability to deform under tensile stress. It is similar to malleability, which characterizes a materials ability to deform under an applied stress. Both of these are plastic properties of materials. While they are often similar, sometimes a materials ductility is independent from its malleability[1]. Ductility is the percentage of plastic deformation right before fracture, where plastic deformation means permanent deformation or change in shape of a solid body without fracture under the action of a sustained force[10]. Materials with low ductility are defined as brittle. Materials with metallic bonds have much higher ductility due to the mobile electrons that tend to deform, rather than fracture. Therefore, the most common ductile materials are steel, copper, gold and aluminum. Ductility is an important property in material science and metal-working industries, where solids are deformed and molded with outside forces. Ductile materials can absorb a large amount of energy before they start to show signs of deformation, whereas brittle materials tend to show deformations and cracks relatively easily.[8]

Environmental factors can also affect the ductility of a material. A temperature increase causes a material to stretch, and thus increases ductility. A temperature decreases leads to brittle and fragile behavior of the material and as such decreases ductility. Generally, low temperatures adversely affect the tensile toughness of many metals. Similarly, pressure can be used to control ductile-brittle effects. Sufficiently large superimposed pressure can convert a generally brittle material into a ductile material.[11]

Metals like aluminum, gold, silver, and copper have a face-centered cubic crystal lattice structure, and most do not experience a shift from ductile to brittle behavior. Other metals, like iron, chromium, and tungsten, have a body-centered cubic crystal structure and experience a sharp shift in ductility. [7]

On the other hand, plastics can also experience variation in ductility through being categorized as a thermoplastic or thermoset. A thermoplastic is a polymer that upon heating becomes moldable and when cooled regains its rigidity through solidifying. Thermoplastics can further be categorized as either amorphous or semicrystalline. In amorphous polymers, the polymer chains are randomly arranged with no long-range ordering and are described as glassy. While in the semicrystalline state, the polymer contains both amorphous and crystalline regions. These crystalline regions involve tightly packed and ordered polymer chains. The molecular differences between these two types of thermoplastics can impact the ductility. For example in amorphous thermoplastics, the ductility is mainly determined by the polymer chain mobility where the chains can glide past each other to accommodate for deformation before fracturing. In semicrystalline materials, the crystalline regions, as they are are ordered in specific directions, allow for easier chain gliding and provide reinforcement to the material. In addition to thermoplastics, thermosets are another polymer category in which the polymer chains are held together by crosslinks. Due to these crosslinks, thermosets tend to be less ductile materials as these linkages don’t allow the material to experience the same amount of percent elongation in comparison to thermoplastics. Therefore, like metals, plastics also experience variation in ductility due to molecular arrangements and morphology.

The Ductile - Brittle Transition Temperature (DBTT) is the temperature at which the fracture energy passes below a predetermined value (typically 40 J) or the point at which the material absorbs 15 ft*lb of impact energy during fracture[7]. The Ductile - Brittle Transition Temperature is an important consideration when determining which material to select, when said material is subjected to mechanical stresses (as shown at https://www.doitpoms.ac.uk/tlplib/BD6/images/graph0.gif). A low DBTT is integral for designs which will need to function in low temperatures [7].

The Ductile to Brittle Transition can also occur when dislocation motion occurs. Dislocation is defined as areas where the atoms are out of position in the crystal structure[12]. The stress required to move a dislocation depends on the atomic bonding, crystal structure, and obstacles. If the stress required to move the dislocation is too high, the metal will fail instead and form cracks or other deformations instead and the failure will be brittle[6].

A Mathematical Model

Mathematically, ductility can be defined as the fracture strain, or the tensile strain along one axis that causes a fracture to occur. Fractures range from brittle fractures (Fig. 1) to fully ductile fractures (Fig. 2), resulting in very different physical appearances associated with the different types. This can be modeled on a stress/strain curve (https://www.nde-ed.org/EducationResources/CommunityCollege/Materials/Graphics/Mechanical/Brittle-Ductile.gif) showing where fracture occurs along the graph.

Quantitatively being able to measure ductility is important with regards to comparing ductility between different materials. Ductility can be measured through two main methods: percent elongation and percent reduction of area[5]. The formulas can be found below: (http://www.engineersedge.com/material_science/ductility.htm)[2]

Percent Elongation = (Final Gage Length - Initial Gage Length) / Initial Gage Length

[math]\displaystyle{ \% elongation = \frac{L_f - L_o}{L_o} \cdot 100 }[/math]

Percent Reduction of Area = (Area of Original Cross Section - Minimum Final Area) / Area of Original Cross Section [math]\displaystyle{ \% Area \ Reduction = \frac{Decrease \ in \ Area}{Original \ Area} }[/math]

A Computational Model

This Ductility Demonstration illustrates the general concept of ductility as a material's ability to undergo some plastic deformation (in this case via stretching a beam), up until some fracture point where the material loses its integrity. On a related note, to learn more about types of plastic deformation, similar to this computational model, see the attached diagram of necking. Necking is a type of deformation often associated with ductile materials. This concept is illustrated in the diagram and additionally within the animation present with the "Ductility Demonstration" computational model!

Connectedness

Stress-Strain Testing Background:

When a material experiences forces such as stress and strain, understanding its performance under such conditions is critical to performance and application. The general calculation for normal stress is taken by dividing the force by the area, as seen below. Similarly, strain can be considered as the elongation over original length.

[math]\displaystyle{ \sigma = \frac{F}{A} }[/math]

[math]\displaystyle{ \varepsilon = \frac{\Delta L}{L} }[/math]

Hooke's Law:

[math]\displaystyle{ \sigma = Modulus \ of \ Elasticity \cdot \varepsilon }[/math]

Modulus of elasticity is a property of the material itself, and is a material’s resistance to elastic deformation (non-permanent). From these equations, the behavior of a material undergoing deformation may be determined.

Based on a material’s modulus of elasticity, a material can be categorized as either “brittle” or “ductile”. A brittle material does not bend, and fractures comparatively quickly. Ductile materials can be easily bent and are comparatively difficult to fracture.

]

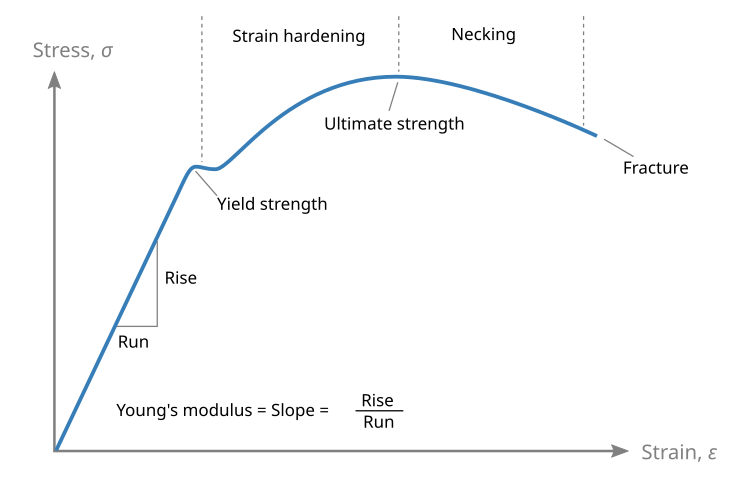
[14] The common points that a ductile material experiences during deformation are identified below. One important characteristic to note is that fracture strength is typically lower than the Ultimate strength of the material. A ductile material is able to withstand additional elongation (strain) even after ultimate strength has been achieved.

[15] This knowledge provides a background to how stress-strain testing is conducted. Different materials are placed inside a stress-strain testing rig. A load is continuously applied to the material until material fracture. A force transducer, combined with a position sensor, allow for the determination of key characteristics. This data allows for further examination using the equations and principles explained above.

Having a solid understanding of ductility and material processes is crucial for material scientists, physicists, mechanical engineers, and several other professions and research disciplines. Understanding the ductility of various materials is incredibly important for having a holistic understanding of a project and for performing proper materials selection and analysis. This is exemplified in materials that have a high applied tensile strength. Significant brittle fractures can cause a great deal of damage and lack of structural integrity. This was seen in Liberty Ships in World War 2, where ships had hull cracks and other significant defects due to the cold temperatures of the water. This ultimately caused the ship's materials to be more brittle in composition. As time progressed, several ships were lost due to brittle fractures, demonstrating the importance of solid materials understanding and the real-world impact that technical oversights can have.[13]

History

Percy Williams Bridgman's findings on tensile strength and material properties led to much of what is known about ductility, including that it is highly influenced by temperature and pressure. These findings led him to win the 1946 Nobel Prize in physics.[4]

]

Ductility

Contents

The Main Idea

A Mathematical Model

A Computational Model

Connectedness

History

See also

Further reading

External links

References

Navigation menu